I’m excited to share that I posted an update to “Fused Extended Two-Way Fixed Effects for Difference-in-Differences With Staggered Adoptions” on arXiv.
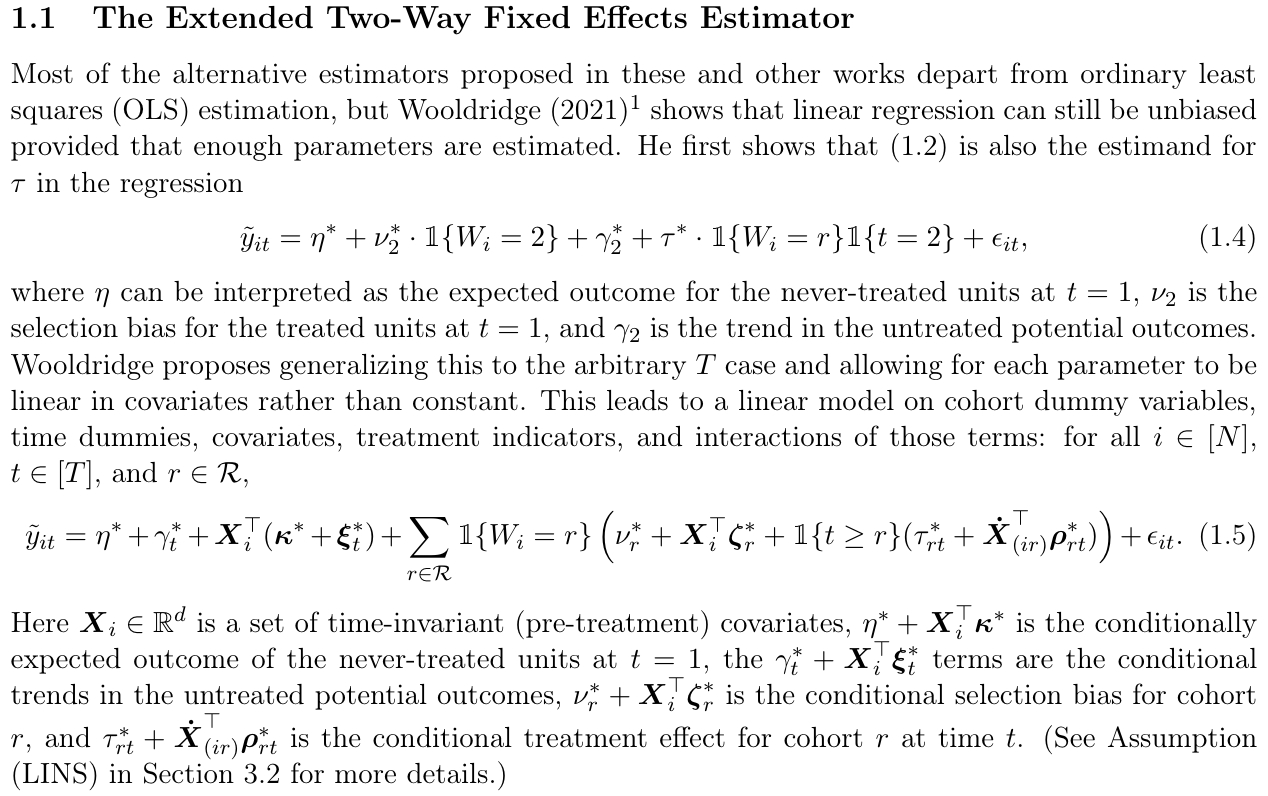
Mainly what’s new in this draft is added theory, but there are some other minor changes. The most notable change to the exposition (in my opinion) is that I revised my introduction of extended two-way fixed effects (Wooldridge 2021) in a way that I think does a better job of conveying the intuition of the estimator than my previous draft.

For an example of the new theory, Theorem D.3 provides a recipe for asymptotic confidence intervals for two broad classes of conditional average treatment effects estimated via FETWFE under conditional parallel trends. As far as I’m aware, FETWFE (along with the original extended two-way fixed effects estimator) is the only difference-in-differences estimator for conditional average treatment effects with theoretical guarantees under staggered adoptions. (Please let me know if you have other references!)
Theorem D.3 applies to broader classes of estimators than I considered in the previous draft. It allows for any function of the estimated generalized propensity scores that is differentiable (via the delta method). Theorems D.1 and D.2 similarly extend the results for the consistency of both conditional and marginal average treatment effects and the asymptotic normality of marginal average treatment effects (respectively) that appeared in the previous draft.
I hope you like the new draft! I intend to release code implementing FETWFE as soon as possible (my code implementing FEWTFE for the simulation studies and empirical application is in a state that isn’t useful for broader application). But it’s hard for me to find time for these things working full time, so I figured for now I’d release what I have ready to share. Whenever I can get the code in a state ready for release, I’ll probably update the draft on arXiv to link to it.